Time Value of Money
Symbols for Interest Formulas:
| N | Number of time periods (typically years but it could be months) |
| i | Interest rate (fixed) over the time period |
| P | Present Value ( Worth / Sum / Amount ) of Future or Annual amounts ($) |
| A | Annual Value ( Worth / Sum / Amount ) of Future or Present amounts ($) |
| F | Future Value ( Worth / Sum / Amount ) of Present or Annual amounts ($) |
The interest rate i and the number of time periods N are applied to find P, A and F. Given I and N and any one of P, A and F, the other two values can be calculated.
Example: $1,000 (present value) is deposited in a bank on the 1st of January 2004 that pays 5% interest on the deposit. What is the future value of this deposit after 5 years?
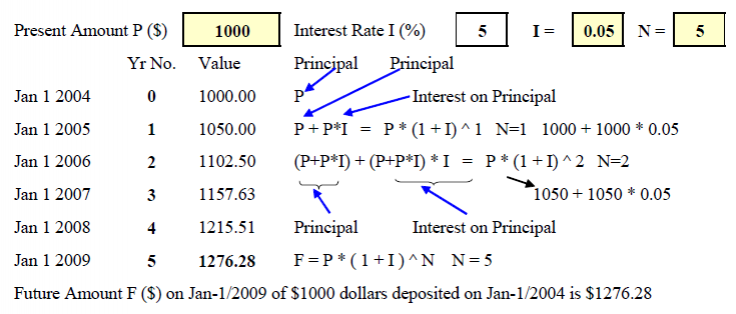
The formula for the future value (F) of a present sum (P) is:
| F = P * ( 1 + I )N | F / P = ( 1 + I )N | F = P * F / P |
The formula for the present value (P) of a future sum (F) is:
| P = F / ( 1 + I )N | P / F = 1 / ( 1 + I )N | P = F * P / F |

Given the interest rate (I) and the number of years (N) use the following formulas:

Example: Future (F) Value of a Present (P) Sum.
$2,000 is deposited into a savings account that earns 6 % interest. What is the future value of this deposit after 10 years?
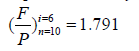 |
F = P * (F/P) = $2,000 * 1.791 = $3,582.00 |
What is the Present (P) value? P = P = $2,000
Example: Future (F) Value of a Annual (A) Sum.
$2,000 will be deposited annually into a savings account that earns 6 % interest. What is the future value of these deposits after 10 years?
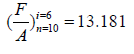 |
F = A * (F/A) = $2,000 * 13.181 = $2,636.20 |
Example: Present (P) Value of a Annual (A) Sum.
$2,000 will be deposited annually into a savings account that earns 6 % interest. What is the present value of these deposits for 10 years?
| P = A * (P/A) = $2,000 * 7.3601 = $2,636.20 |
Example: Present (P) Value of a Future (F) Sum.
A car dealer agrees to let you pay $20,000 at the end of 10 years at the future value of dollar in 10 years for a car that you can start using now. What is the cost of this car today?
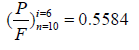 |
P = F * (P/F) = $20,000 * 0.5584 = $11,168.00 |
Example: Annual (A) Value of a Future (F) Sum.
The parents of an 8 year old child estimate that they will need $80,000 (future dollars) in 10 years for the child's college education. How much must they deposit (in present dollar value) each year for the next 10 years at 6% interest to have $80,000 in the bank 10 years from now?
| A = F * (A/F) = $80,000 * 0.07587 = $6,069.60 |
Note that $6,069.60 times 10 year is $60,696 and not $80,000. The first years deposit earns interest for 10 years, the second years deposit earns interest for 9 years, etc.
Example: Annual (A) Value of a Present (P) Sum.
The loan on a car is $20,000 and the payment period is 10 years. What is the annual payment?
| A = P * (A/P) = $20,000 * 0.13587 = $2,717.40 ($226.45/month??) |
Note if the payments are paid monthly then the principal is reduced every month and the monthly payment is $222.05 which is less than $2,717.40 / 12 = $226.45.
What is the total cash payment and how much was paid in interest?
Cash paid = $2,717.40 * 10 years = $27,174.00. Interest = $27,174 - $20,000 = $7,174.
Life Cycle Cost Analysis (LCCA)
Life Cycle Cost Analysis (LCCA) is a design process for controlling the initial and the future cost of developing and owning a building. LCCA can also be an effective tool for evaluation of existing building systems and replacing those systems. LCCA can be used to evaluate the cost of a full range of projects, from an entire site complex to a specific building system component.
Life Cycle Cost (LCC) is the total discounted (present value) dollar cost of owning, operating, maintaining, and disposing of a building or a building system over a period of time. The LCC equation can be broken down into the following three variables:
- Costs of ownership
- Period of time over which the costs are incurred
- Discount rate that is applied to future costs to equate them with present day costs.
Initial & Future Expenses
The first component in a LCC equation is cost. There are two major cost categories by which projects are to be evaluated in a LCCA. They are:
- Initial Expenses (First Costs)
- Future Expenses (operation, maintenance, repair, energy, replacement)
Initial Expenses are all costs incurred prior to occupation of the facility. Future Expenses are all costs incurred after occupation of the facility.
Defining the exact costs of each expense category can be somewhat difficult since, at the time of the LCC study, nearly all costs are unknown. However, through the use of reasonable, consistent, and well-documented assumptions, a credible LCCA can be prepared. If costs in a particular cost category are equal in all project alternatives, they can be documented as such and removed from consideration in the LCC comparison.
Study Period (N)
The second component of the LCC equation is time. The study period is the period of time over which ownership and operations expenses are to be evaluated. Typically, the study period can range from five to fifty years, depending on owner's preferences, the stability of the user's program, and the intended overall life of the facility.
For example, a speculative building developer intends to sell the building for profit after it as appreciated in value for ten years. So the developer is interested in a ten year LCCA. Some owner occupiers might plan on occupying the building indefinitely. They would be interested in the return in their investment over a twenty year period. It is quite common to look at LCCA for different periods. While the length of the study period is often a reflection of the intended life of a facility, the study period is usually shorter than the intended life of the facility.
The National Institute of Science & Technology (NIST) breaks the study period into two phases: the planning/construction period and the service period. The planning/construction period is the time period from the start of the study to the date the building becomes operational (the service or occupied date). The service period is the time period from date the building becomes operational to the end of the study.
Sometimes the planning and construction period can take several years to complete. To remove the uncertainty regarding the appropriate length of the planning/construction period and to simplify the LCC calculation, some analysis will assume that all initial costs will be incurred in the base year of the study. Thus, all initial costs will be entered into the LCCA at their full value. For government buildings, the typical recommended study period for LCCA is twenty to twenty-five years.
Inflation
The actual dollar value of costs at different points in time cannot be compared directly because of the effect of inflation. Inflation is the loss in purchasing power due to an increase in the price level of goods and services without a corresponding increase in the quantity or quality these goods and services. Examples of economic factors that cause inflation are: (1) deficit government spending where the government prints or mints more money to cover the costs of their projects and (2) every worker in the country gets a 10% raise in wages without an increase in production which results in a corresponding increase in the cost of goods and services.
Discount Rate (D)
The third component in the LCC equation is the discount rate. The discount rate is defined as the "the rate of interest reflecting the investor's time value of money." Basically, it is the interest rate that would make an investor indifferent as to whether he received a payment now or a greater payment (due to inflation) at some time in the future. The investor's financial status remains the same over the years and there is no gain or loss.
The NIST takes the definition of discount rates a step further by separating them into two types:
- Real discount rates
- Nominal discount rates
The difference between the two is that the real discount rate excludes the rate of inflation and the nominal discount rate includes the rate of inflation. This is not to say that real discount rates ignore inflation. Since alternatives are being compared, the use of real discount rate eliminates the complexity of accounting for inflation within the present value equation. The use of either discount rate in its corresponding present value calculation derives the same result.
Obviously, as the economics of the world around us change, so to does the discount rate. To establish a standard discount rate to be used in LCCA, the department has adopted the US Department of Energy's real discount rate. This rate is updated and published annually in the Energy Price Indices and Discount Factors for Life-Cycle Cost Analysis - Annual Supplement to NIST Handbook 135. The rate will also be updated annually in the department's LCC spreadsheet tool, available on the department's web site.
X = inflation Rate. Dr = Real Discount Rate. Dn = Nominal Discount Rate.
Rates are fractions (percent/100).
Constant-Dollars
Just as discount rates can be defined as either real or nominal, so too can costs. NIST defines constant-dollars as "dollars of uniform purchasing power tied to a reference year and exclusive of general price inflation or deflation." The NIST defines current dollars as "dollars of non-uniform purchasing power, including general price inflation or deflation, in which actual prices are stated."
When using the real discount rate in present value calculations, costs must be expressed in constant dollars. Likewise, when using the nominal discount rate in present value calculations, costs must be expressed in current-dollars. In the rare case that the inflation rate is zero, constant-dollars are equal to current-dollars and the real discount rate is equal to the nominal discount rate.
In practice, the use of constant-dollars simplifies LCCA. For example, suppose one wants to evaluate roofing products over a 30-year period. However, one roofing product must be replaced after 20 years. How much will the replacement of the roof cost in 20 years? By using constant dollars (use today’s value of the roof), the guesswork of estimating the escalation of labor and material costs is eliminated. The future constant dollar cost (excluding demolition) to install a new roof in 20 years is the same as the initial cost to install the roof. Any change in the value of money over time will be accounted for by the real discount rate.
Present Value (PV)
To accurately combine initial expenses with future expenses, the present value of all expenses must first be determined. NIST defines present value as "the time-equivalent value of past, present or future cash flows as of the beginning of the base year."
The present value calculation uses the discount rate and the time a cost was or will be incurred to establish the present value of the cost in the base year of the study period. Since most initial expenses occur at about the same time, initial expenses are considered to occur during the base year of the study period. Thus, there is no need to calculate the present value of these initial expenses because their present value is equal to their actual cost.
The determination of the present value of future costs is time dependent. The time period is the difference between the time of initial costs and the time of future costs. Initial costs are incurred at the beginning of the study period at Year 0, the base year. Future costs can be incurred anytime between Year 1 and Year 20. The present value calculation is the equalizer that allows the summation of initial and future costs.
Future costs can be broken down into two categories:
- One-time costs
- Recurring costs
One-time costs are costs that do not occur ever year over the span of the study period. Most replacement costs are one-time costs. For example, the study period is 25 years and the life of the equipment is 15 years. Recurring costs are costs that occur ever year over the span of the study period. Most energy, operating and maintenance costs are recurring costs.
To simplify the LCCA, all recurring costs are expressed as annual expenses incurred at the end of each year and one-time costs are incurred at the end of the year in which they occur.
Residual Value
Residual value is the net worth of a building at the end of the LCCA study period. Unlike other future expenses, an alternative's residual value can be positive or negative.
A negative residual value indicates that there is value associated with the building at the end of the study period. For example the System or Plant can be sold as used equipment. The remaining value is a tangible asset of building ownership and should be included in the LCCA.
A positive residual value indicates that there are disposal costs associated with the building at the end of the study period. It is a future expense such as disposal of the equipment or demolition of the structure. Zero residual value indicates that there is no value or cost associated with the building at the end of the study period.
Initial Investment Costs (First Costs)
The first step in the completion of the LCCA of a project alternative is to define all the initial investment costs of the alternative. Initial investment costs are costs that will be incurred prior to the occupation of the facility. All initial costs are to be added to the LCCA total at their full value. The level of detail of these costs should be commensurate with the level of project detail.
Operating Costs
The second step in the completion of the LCCA of a project alternative is to define all the future operating costs of the alternative. The operating costs are annual costs, excluding maintenance and repair costs, involved in the operation of the facility. Most of these costs are related to building utilities and custodial services. All operating costs are to be discounted to their present value prior to addition to the LCCA total.
Operating costs that are not directly related to the building should usually be excluded from the LCCA. An example of a cost that should be excluded is the cost of office materials. While it is an annual operating expense, it has nothing to do with the operation of the building but is rather, a function of the building user.
Maintenance & Repair Costs
The third step in the completion of the LCCA of a project alternative is to define all the future maintenance and repair costs of the alternative. Maintenance costs are scheduled costs associated with the upkeep of the facility. An example of a maintenance cost is the cost of an annual roof inspection and caulking of the building's roof penetrations. This task is a scheduled event that is intended to keep the building in good condition.
Repair costs are unanticipated expenditures that are required to prolong the life of a building system without replacing the system. An example is the repair of a broken window. This is an unscheduled event that does not entail replacement of the entire window unit, merely the replacement of the broken pane.
Some maintenance costs are incurred annually and others less frequently. Repair costs are by definition unforeseen so it is impossible to predict when they will occur. Maintenance and repair costs should be treated as annual costs. All maintenance and repair costs are to be discounted to their present value prior to addition to the LCCA total.
The maintenance and repair costs for all options may not be equal. For example equipment with lower first costs will require more maintenance and repair. It is possible that one alternative might be more susceptible to damage than others.
Replacement Costs
The fourth step in the completion of the LCCA of a project alternative is to define all the future replacement costs of the alternative. Replacement costs are anticipated expenditures to major building system components that are required to maintain the operation of a facility. All replacement costs are to be discounted to their present value prior to addition to the LCCA total.
Replacement costs are typically generated by replacement of a building system or component that reaches the end of its useful life before the time period of the LCCA. An example of a replacement cost is the replacement of a boiler. A boiler has a life expectancy that is shorter than that of the building it serves. At some point it will fail and require replacement to keep the facility operational.
An example is the evaluation of two roofing alternatives, a metal roof and a composition shingle roof. The shingle roof has a life span of 20 years where as the metal roof is expected to last 40 years. In a LCCA over a 30-year study period the shingle roof will have to be replaced, thus incurring replacement costs. The metal roof will not require replacement; thus no replacement costs will be incurred during the 30 year LCCA period.
If the constant-dollar approach to LCCA is used, the cost to replace a building component in the future will be the same as the current cost of the building component plus demolition costs.
Residual Value
The fifth step in the completion of the LCCA of a project alternative is to define the residual value of the alternative. Residual value, as defined earlier, is the net worth of a building or building system at the end of the LCCA study period. This is the only cost category in a LCCA where a negative value, one that reduces cost, is acceptable. The residual value of a facility or building system is especially important when evaluating project alternatives that have different life expectancies.
DEFINITIONS
Constant-Dollars: dollars that have uniform purchasing power over time and that are not affected by general price inflation or deflation.
Current-Dollars: dollars that do not have uniform purchasing power over time and that are affected by general price inflation or deflation.
Discount Rate: the rate of interest that balances an investor's time value of money.
Initial Investment Cost: any cost of creation of a facility prior to its occupation.
Life Cycle Cost: a sum of all costs of creation and operation of a facility over a period of time.
Life Cycle Cost Analysis: a technique used to evaluate the economic consequences over a period of time of mutually exclusive project alternatives.
Maintenance Cost: any cost of scheduled upkeep of building, building system, or building component.
Nominal Discount Rate: a discount rate that includes the rate of inflation. Operating Cost: any cost of the daily function of a facility. Present Value: the current value of a past or future sum of money as a function of an investor's time value of money
Real Discount Rate: a discount rate that excludes the rate of inflation.
Repair Cost: any cost of unscheduled upkeep of a building system that does not require replacement of the entire system
Replacement Cost: any cost of scheduled replacement of a building system or component that has reached the end of its design life.
Residual Value: the value of a building or building system at the end of the study period. Study Period: the time period over which a Life Cycle Cost Analysis is performed.
Electric Rates
Base Charge:
This is a monthly service charge. I covers the cost of providing the service. This charge applies even if no power is used.
Energy (Consumption) Charge:
This a charge for the amount of electricity used. Most utility companies have a rate schedule
Editor's picks for more information on time value of money:
Future Value - Walk through a DCF

